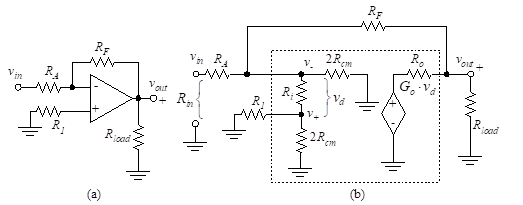
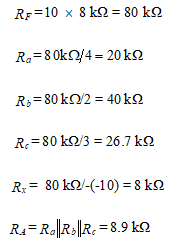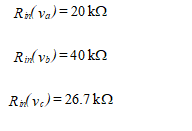Inverting Amplifier-TINA and TINACloud

Figure 36(a) illustrates an inverting amplifier. Figure 36(b) shows the equivalent circuit using the op-amp model developed earlier in this chapter.

Figure 36 – Inverting amplifier

8.1 Input and Output Resistance

Figure 36(b) is reduced to Figure 37(a) if we let,

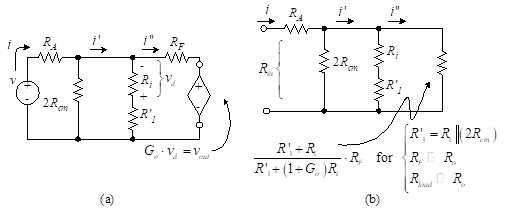
Figure 37 – Simplified inverting amplifier model

It is reasonable to assume that these inequalities apply because, were they not true, the output would load the input and the gain would be reduced.

A voltage-divider relationship can be used to yield

(71)

and a loop equation yields

(72)

The input resistance, R_in, is obtained from Figure 37(b), where we have replaced the dependent source with an equivalent resistance. The value of this resistor is v_–/i” which is found from Equation (72) . For large G (i.e., ), the rightmost resistance in Figure 37(b) is approximately zero, and .

The output resistance of the inverting amplifier is the same as that of the non-inverting amplifier. Thus,

(73)

8.2 Voltage Gain

We use the equivalent circuits of Figure 36(b) and Figure 37(a) to determine the voltage gain. The inverting input gain, A_–= v_out/v_in, is obtained from the circuit of Figure 37(a) by again making the same assumptions that we made in finding the output resistance.

These assumptions reduce the circuit to that shown in Figure 38(a), where we have changed the voltage source in series with a resistance to a current source in parallel with a resistance. The resistors can then be combined to yield the circuit of Figure 38(b). Finally, the current source is converted back to the voltage source to yield the simplified circuit of Figure 38(c).

The loop equation for this circuit is given by

(74)

Since v_out = G_ov_d, the inverting voltage gain is

(75)

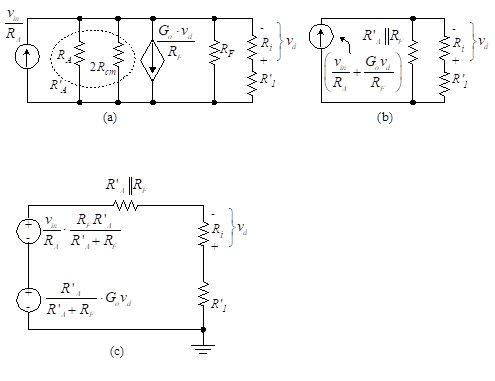
Figure 38 (parts a, b, c)- Inverting input gain

We can verify this result relative to the gain of the ideal op-amp by making the approximations: R_A << 2R_cm and G >> 1. Then

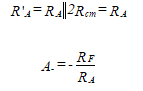
(76)

This is the same as the result found earlier for the simplified model.

8.3 Multiple-Input Amplifiers

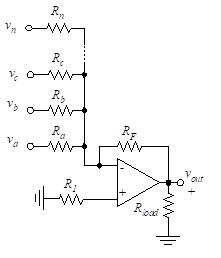
(39)

If the voltages v_a, v_b, … , v_m are applied to the summing junction (inverting input to op-amp) through resistors R_a, R_b, …, R_m, respectively, as shown in Figure 39, the output voltage is

(77)

To achieve bias balance, we choose

(78)

Let us define

(79)

The output resistance is then

(80)

Suppose now that only two inputs are used. The output voltage is then

(81)

The input resistance at v_a is approximately equal to R_a, and the input resistance at v_b is approximately R_b. We can make this circuit a unity-gain two-input summer with an output voltage of

(82)

by setting R_F = R_a = R_b. The resistance from the non-inverting input terminal to ground is chosen to achieve bias balance. Thus, R₁ = R_F/3, and we have